Poker Bankroll Risk Of Ruin Calculator

The bankroll required to have a one percent risk of ruin is about 20 percent higher than the bankroll for a five percent risk of ruin. The bankroll as a percentage of the total dollars played decreases as the number of plays increases. SINGLE VERSUS MULTIPLE PLAY WITH SAME BET AMOUNT. Nov 18, 2013 Risk of Ruin Simulator and Calculator I built a function that works in a free program (called R - you can download it at www.r-project.org ) for simulating your risk of ruin. I have pasted the function below, but first let me explain a few things.
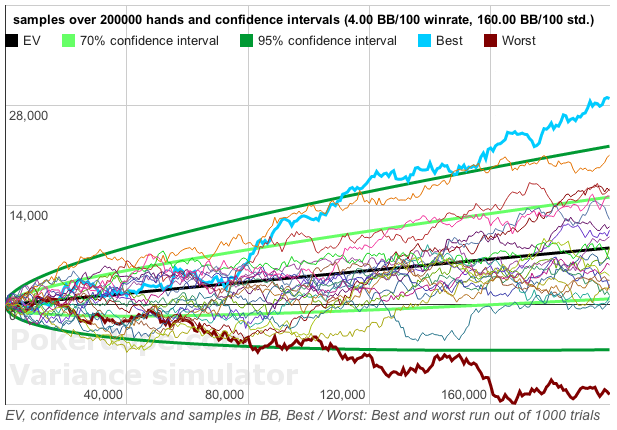
Risk of ruin is a concept in gambling, insurance, and finance relating to the likelihood of losing all one's investment capital[1] or extinguishing one's bankroll below the minimum for further play. For instance, if someone bets all their money on a simple coin toss, the risk of ruin is 50%. In a multiple-bet scenario, risk of ruin accumulates with the number of bets: each repeated play increases the risk, and persistent play ultimately yields the stochastic certainty of gambler's ruin.
- 1Finance
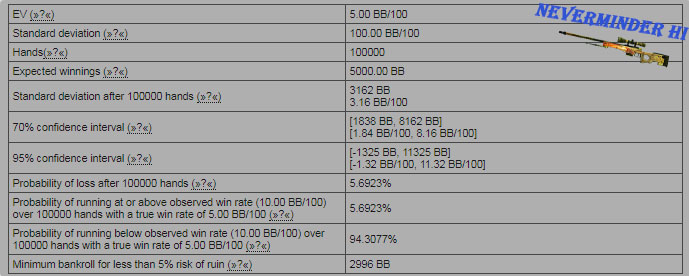
Your optimal bet size is 25% of your bankroll. (Now, find a casino stupid enough to offer those odds!) Of course, you can see practical the practical value of Kelly betting when it comes to things with discrete results and obvious probabilities - say pot odds in a poker hand. Given a winning poker player with win rate of $30/hour, standard deviation of $600 and bankroll of $15000: Risk of ruin = e ^ (-2.30.15000/ (600^2)) Risk of ruin = 8.208500% Although this is a precise answer given to 6 decimal places it is only an approximation and probably would be rounded up to give a 10% risk of ruin in practice. Risk of ruin has primarily been used to determine bankroll size needed for negative expectation games. It could be used for poker but you would have to know a few things. Your expected edge in the game (Could only accurately be determined after tens or hundreds of thousands of hands played to have significant mathematical certainty.).
Risk Of Ruin Calculator Blackjack
Finance[edit]
Risk of ruin for investors[edit]
Two leading strategies for minimising the risk of ruin are diversification and hedging. An investor who pursues diversification will try to own a broad range of assets – they might own a mix of shares, bonds, real estate and liquid assets like cash and gold. The portfolios of bonds and shares might themselves be split over different markets – for example a highly diverse investor might like to own shares on the LSE, the NYSE and various other bourses. So even if there is a major crash affecting the shares on any one exchange, only a part of the investors holdings should suffer losses. Protecting from risk of ruin by diversification became more challenging after the financial crisis of 2007–2010 – at various periods during the crises, until it was stabilised in mid-2009, there were periods when asset classes correlated in all global regions. For example, there were times when stocks and bonds [2] fell at once – normally when stocks fall in value, bonds will rise, and vice versa. Other strategies for minimising risk of ruin include carefully controlling the use of leverage and exposure to assets that have unlimited loss when things go wrong (e.g., Some financial products that involve short selling can deliver high returns, but if the market goes against the trade, the investor can lose significantly more than the price they paid to buy the product.)
The probability of ruin is approximately
Poker Bankroll Management
- ,
where
Northern california casinos with roulette tables. River Rock Roulette is the newest sensation to hit Sonoma County and it's exclusive to River Rock Casino! The layout and payouts are the same as traditional roulette, only the cards represent the winning number instead of a ball. Get into the action by picking a single lucky number, splits, or small combinations of your favorite numbers.
for a random walk with a starting value of s, and at every iterative step, is moved by a normal distribution having mean μ and standard deviation σ and failure occurs if it reaches 0 or a negative value. For example, with a starting value of 10, at each iteration, a Gaussian random variable having mean 0.1 and standard deviation 1 is added to the value from the previous iteration. In this formula, s is 10, σ is 1, μ is 0.1, and so r is the square root of 1.01, or about 1.005. The mean of the distribution added to the previous value every time is positive, but not nearly as large as the standard deviation, so there is a risk of it falling to negative values before taking off indefinitely toward positive infinity. This formula predicts a probability of failure using these parameters of about 0.1371, or a 13.71% risk of ruin. This approximation becomes more accurate when the number of steps typically expected for ruin to occur, if it occurs, becomes larger; it is not very accurate if the very first step could make or break it. This is because it is an exact solution if the random variable added at each step is not a Gaussian random variable but rather a binomial random variable with parameter n=2. However, repeatedly adding a random variable that is not distributed by a Gaussian distribution into a running sum in this way asymptotically becomes indistinguishable from adding Gaussian distributed random variables, by the law of large numbers.
Risk Of Ruin Calculator Poker
Financial trading[edit]
The term 'risk of ruin' is sometimes used in a narrow technical sense by financial traders to refer to the risk of losses reducing a trading account below minimum requirements to make further trades. Random walk assumptions permit precise calculation of the risk of ruin for a given number of trades. For example, assume one has $1000 available in an account that one can afford to draw down before the broker will start issuing margin calls. Also, assume each trade can either win or lose, with a 50% chance of a loss, capped at $200. Then for four trades or less, the risk of ruin is zero. For five trades, the risk of ruin is about 3% since all five trades would have to fail for the account to be ruined. For additional trades, the accumulated risk of ruin slowly increases. Calculations of risk become much more complex under a realistic variety of conditions. To see a set of formulae to cover simple related scenarios, see Gambler's ruin. Opinions among traders about the importance of the 'risk of ruin' calculations are mixed; some[who?] advise that for practical purposes it is a close to worthless statistic, while others[who?] say it is of the utmost importance for an active trader to be aware of it.[3][4]
See also[edit]
Poker Risk Of Ruin Calculator

- Absorbing Markov chain (used in mathematical finance to calculate risk of ruin)
- Fat-tailed distribution (exhibits the difficulty and unreliability of calculating risk of ruin)
- St. Petersburg paradox (an imaginary game with no risk of ruin and positive expected returns, yet paradoxically perceived to be of low investment value)
Notes and references[edit]
- ^'Risk of Ruin (Forex Glossary)'. Financial Trading Journal. Retrieved April 26, 2012.
- ^Though US treasuries were generally an exception, except on the very worst days their value generally rose, as part of the 'Flight to safety'.
- ^Trading Risk: Enhanced Profitability through Risk Control Kenneth L Grant (2009)
- ^The trading game Ryan Jones (1999)
Further reading[edit]

Video Poker Risk Of Ruin Calculator
- Dickson, David C. M. (2005). Insurance Risk And Ruin. Cambridge University Press. Retrieved April 26, 2012.ISBN0521846404
- Powers, Mark J. (2001). Starting Out in Futures Trading. McGraw-Hill. pp. 52–55. Retrieved April 26, 2012.ISBN0071363904
- Baird, Allen Jan (2001). Electronic Trading Masters: Secrets from the Pros!. John Wiley & Sons, Inc. pp. 30–32. Retrieved April 26, 2012.ISBN0471401935
By John Grochowski
My friend Mark isn’t a casino regular, but he likes to play a little video poker now and then. His goal is just to have a good time and stay in action for a couple of hours.
“Do you have a guide to how much cash I need to last a couple of hours?” he asked.
I showed him the bankroll calculator on Video Poker for Winners software, and assumed expert play for 1,000 hands --- about two hours play for an average player.
First up was Jacks or Better on three pay tables --- the full-pay 9-6 game, paying 9-for-1 on full houses and 6-for-1 on flushes, which returns 99.54 percent with expert play; the 8-5 game (97.30 percent); and the 7-5 game (96.15 percent) that’s becoming all too common on quarter games.
Jacks or Better is the least volatile of common video poker games, a game that’s designed to keep you in your seat. There are no big four-of-a-kind bonuses that are going to make your day. All quads pay 125 coins for a five-coin wager. But the 2-for-1 payoff on two pairs packs a different kind of wallop, one that will keep you going for extra chances at the bigger pays.
The average loss for two hours of betting $1.25 a hand on a quarter machine is $5.75 with a 9-6 pay table, $34.75 at 8-5 and $48.12 at 7-5 --- which ought to tell you why I’m always harping on finding the best pay tables. In the days when each video poker machine had just one game --- no touching the screen to try a different game --- I once found a long row of 18 machines that alternated between 9-6 and 8-5 pay tables. There were as many players at the low-payers as at the 9-6ers. Ugh.
The required bankroll is much higher than the average loss if you want to give yourself enough for a 5 percent risk of ruin --- a 95 percent chance of surviving two hours without losing it all. That takes $165 on 9-6 Jacks, $185 at 8-5 and $195 at 7-5.
Your chances of having a winning session after two hours are 34.54 percent at 9-6, 22.35 percent at 8-5 and 17.19 percent at 7-5. Settling for a 7-5 pay table instead of 9-6 cuts your chances of winning in half.
Then I checked probably the most popular video poker game: Double Double Bonus Poker. With a 9-6 pay table, it’s a 98.98 percent return, $12.75 average loss in two hours on a quarter machine, with a $300 bankroll for a 5 percent risk of ruin and a 35.46 percent chance of a winning session. On the 8-5 version that’s become all too common, the payback percentage falls to 96.79 percent, average two-hour loss increases to $40.12, the bankroll requirement rises to $320, and the chance of a winning session drops to 30.75 percent.
Double Double Bonus Poker is the more volatile game, with more of its payback concentrated into relatively rare four-of-a-kind hands. Most quads pay 250 for a five-coin wager, and the reward rises to 400 on four 2s, 3s or 4s; 800 if those low quads are accompanied by an Ace, 2, 3 or 4 kicker; 800 on four Aces; and 2,000 on four Aces with a 2, 3 or 4 kicker. The two-pairs return is reduced to 1-for-1 ---- you just get your money back.
That’s why Double Double Bonus bankroll requirements are higher than in Jacks or Better. But in any game, cuts in the pay table slash your chances of winning. Be wary.
LONGER SESSIONS: Two-hour sessions are extremely volatile. Just about anything can happen in any session as short as a couple of hours. But I’ve had many a two-hour session back when that was the length of a riverboat casino cruise, and still often go to a local casino to play for a couple of hours and have lunch or dinner.
But what if you’re going to play longer? What if you’re going on an overnight stay and figure to get in, say, 10 hours of play? Do you have to multiply two-hour bankroll requirements by five?
No, you don’t. Longer sessions smooth things out a bit. For 10 hours of quarter play on 9-6 Jacks or Better, the bankroll for a 5 percent risk of ruin doesn’t quintuple from $165 to $825. Instead, it’s less than tripled, at $450, while the bankroll requirement for 8-5 Jacks rises to $570.
On the more volatile Double Double Bonus Poker, that $300 bankroll for a 5 percent risk or ruin for two hours rises to $885. That’s a big chunk of cash, but at least it’s not the $1,500 you get when multiplying the $300 by five. On the 8-5 version, the bankroll needed for 10 hours is $1,010, and that’s one reason I just won’t play 8-5 Double Double Bonus Poker.
Risk Of Ruin Calculator Video Poker
Bankroll For Live Poker
John Grochowski writes about casino games and the gambling industry in his weekly 'Gaming' column, which is syndicated in newspapers and Web sites across the United States. John is also the author of six books on casinos and casino games.



